Elementary Transformations Applied to

Quadratic Functions

By

Alex Moore

In this exploration we will investigate the elementary transformations of quadratic equations, that is, equations of the form f(x) = a(x^2) + bx + c for constants a,b, and c. For our purposes we will assume that a, b, and c are real constants. By elementary transformations we mean given a function f(x) we can transform the graph of f in nine ways: for any positive number s,

1) The identity transformation, f(x);

2) Reflection across the y-axis, f(-x);

3) Reflection across the x-axis, -f(x);

4) Vertical stretch or compression, s*f(x);

5) Horizontal stretch or compression, f(s*x)

6) Shifting the graph to the right s units, f(x-s);

7) Shifting the graph to the left s units, f(x+s);

8) Shifting the graph vertically up s units, f(x)+s;

9) Shifting the graph vertically down s units, f(x)-s.

This exploration will give examples as to how the transformation affects quadratics and after experimenting we will combine transformations. As a starting example we will consider the quadratic function f(x)=2(x^2)+3x-4. Below are transformations of type 6) and 7) above for the s=8,4,0,-4, and -8.

While these examples are not proof that these transformations do as I mentioned above they do provide convincing evidence to support my claim. Let us now examine transformations of the form in 8) and 9) for the same values of s.

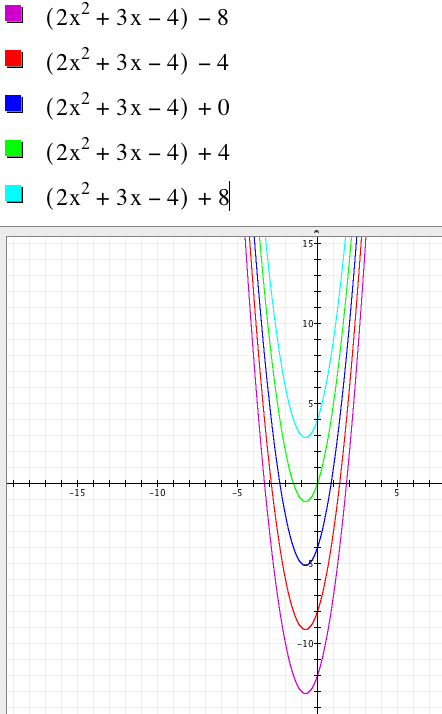
Once again this is very convincing evidence to the claims made above. Now we will test transformations of the form in 4) and 5). We will let s=3, 2, 1, ½ , and ¼.

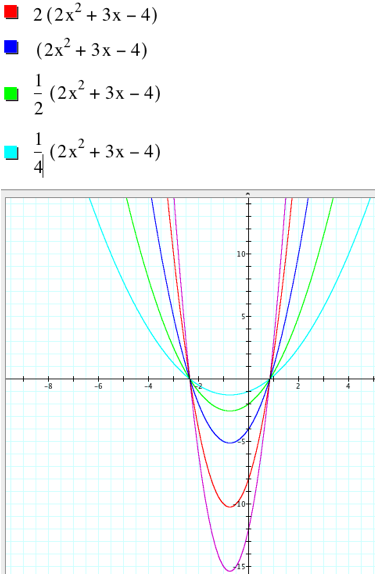
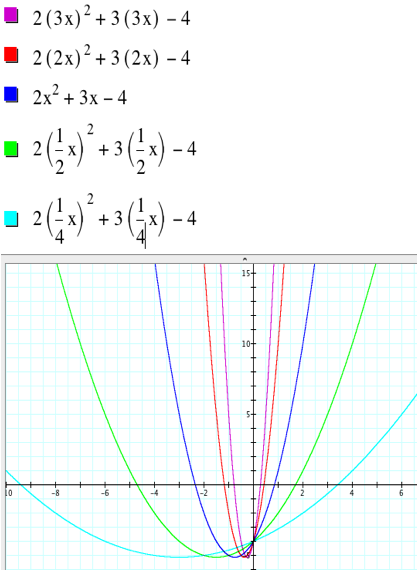
Notice how in the transformations s*f(x) the x-intercepts remain the same while the y-intercept changes, while with transformations of the form f(s*x) the y-intercept remains constant while the x-intercepts change. This is an easily proven fact.

Proposition: Suppose f(x) is a function and r is a real number such that f(r)=0. Then g(x)=s*f(x) is a function with g(r)=0 for all real s.

Proof: Suppose f(r)=0. Define g(x)=s*f(x) for any real number s we have g(r)=s*f(r)=s*0=0.

Clearly the y-intercepts do not stay the same as the examples above show.

Proposition: Suppose f(x) is a function and f(0)=y for some real y. Then g(x)=f(s*x) is a function with g(0)=y for all real s.

Proof: Suppose f(0)=y and define g(x)=f(s*x). Then g(0)=f(s*0)=f(0)=y since s*0=0 for all real s.

We have now proven that vertical stretches and compressions preserve the x-intercepts and that horizontal stretches and compressions preserve the y-intercept. Now we Demonstrate the last two types of nontrivial transformations; types 2) and 3).

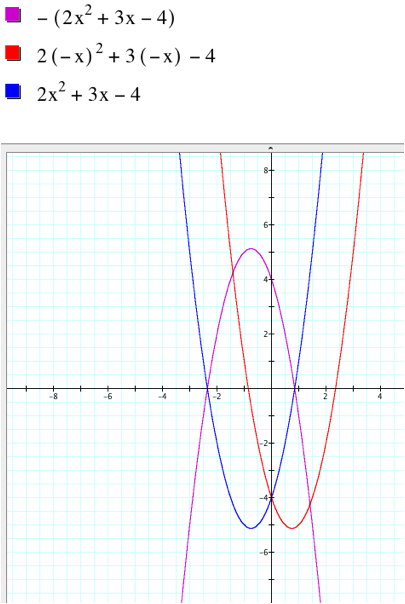
Let us continue to work with our basic example f(x)=2(x^2)+3x-4. Suppose we wanted to transform the graph of f(x) so that the vertex rest in the second quadrant rather than the third? How about if we wanted the graph to be concave down but keep the same vertex? These are simply straight forward applications of our transformations, as shown below:

To move the vertex to the second quadrant we simple shift left 5 units and then shift up 8 units (this is only one possible solution to the problem). For the second task we first note that we can rewrite f(x) as f(x)=2((x+ ¾ )^2)-(41/8). This means that when we reflect across the x-axis our new y-coordinate of the vertex will be 41/8 and we must shift back down to -41/8. This is a total distance of 41/8 –(-41/8)=41/4=10.25 and so we have our desired result. As these two simple examples demonstrate we can control our quadratic functions with absolute precision by simply using these elementary transformations.